ORIGO Education was founded in 1995 on the premise that mathematics should be taught conceptually. But what does teaching conceptually mean, and why should math be taught that way?
In elementary school, mathematics has always relied heavily on students remembering procedures and rules, with the occasional trick to help them calculate correct answers. With this teaching method, the focus is on the acquisition of skills where accuracy and speed are rewarded, rather than understanding the concepts behind the math. Students learned how to manipulate symbols, rather than hands-on resources, and there is certainly no need for discussion or collaboration. Quite simply, if you follow the rule, you will get the right answer, and everyone will be happy!
Fortunately, educational research over the past few decades has provided the necessary data to shift the focus from routinely learning mathematics skills to understanding mathematical concepts—and it’s for the better!
What does it mean to teach mathematics conceptually?
As the name suggests, a conceptual approach requires a greater focus on teaching the concepts or ideas of mathematics and making sure students understand the connections between them. For example, in the past, students would learn the meaning of the equivalence and subtraction symbols as soon as possible, since this was necessary for writing equations or applying the written subtraction algorithm. They would then use this skill to complete drills and exercises in the hope that this would help them memorize their basic subtraction facts.
Now, we spend time ensuring students understand the ‘idea’ of subtraction and the fact that there are in fact three different types. In doing so, they learn that in all cases there is always a total, a known part, and a missing part. The missing part could be the amount left (take-from subtraction), the other part (unknown-addend subtraction), or the difference (comparison subtraction).
As students learn these types of subtraction situations, they make connections between these new ideas and the concept of addition which they would already know. In simple terms, they learn how a subtraction can undo addition and vice versa. When students understand the concepts behind subtraction and the connection with addition, they then practice the skill of subtraction by learning their basic facts.
No doubt there are differing views on what constitutes conceptual teaching, but as I understand it, and as ORIGO teaches it, there must be visual models and the use of mathematical language and discourse.
Visual Models
When teaching for understanding, students need visual representations of mathematical concepts, ranging from discrete physical objects or pictures to continuous models that have attributes of length, distance, and area. A carefully sequenced mathematics program will utilise these representations across all the elementary grades and further into middle school.
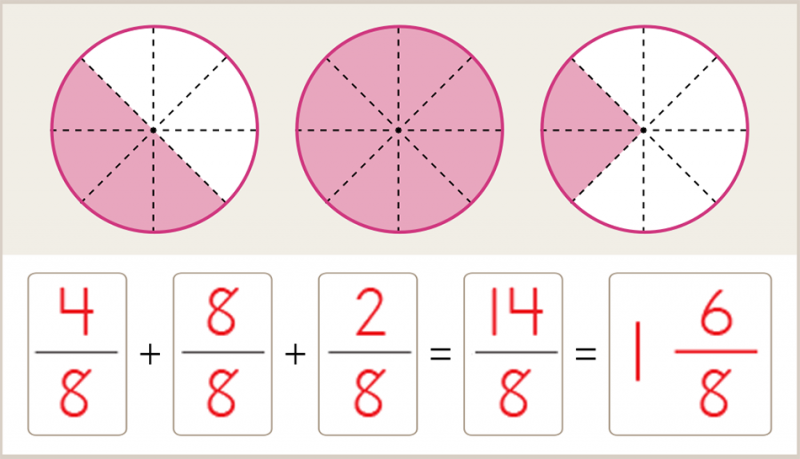
In early grades, students learn the concept of fractions (for example, one-half) using hands-on experiences with area and length models. Later, they discover they can find one half of a set of objects and they can mark a point on a number line that is half the distance from 0 to 1. As students progress to higher grades, they continue to use number lines and area models to learn about fractions greater than 1, and again as they begin to understand the meaning behind operating with fractions.
Language and Discourse
A conceptual approach encourages students to share with and explain to their peers the connections they see between and among mathematical concepts. This provides a necessary opportunity for students to build their understanding of concepts by discussing and justifying their own thinking and by evaluating other students’ thinking in constructive and supportive settings.
Most importantly, students are encouraged to apply these understandings and build upon their knowledge to think and reason mathematically in problem-solving situations that are rich and meaningful to them in their everyday life.
Why teach mathematics conceptually?
As educators, it is our responsibility to prepare students for their future. Is there real value in teaching students’ skills or ‘procedures’ such as the long division algorithm? If we don’t actually use it today, it is even more unlikely that will use it tomorrow.
We now know that higher-order thinking and creative problem-solving are the new in-demand skills for the 21st century. To build these skills, students need time to develop deep understandings of concepts and the connections between them. In short, we must give students visual models and the opportunity to discuss their thinking, so they can learn mathematics conceptually.
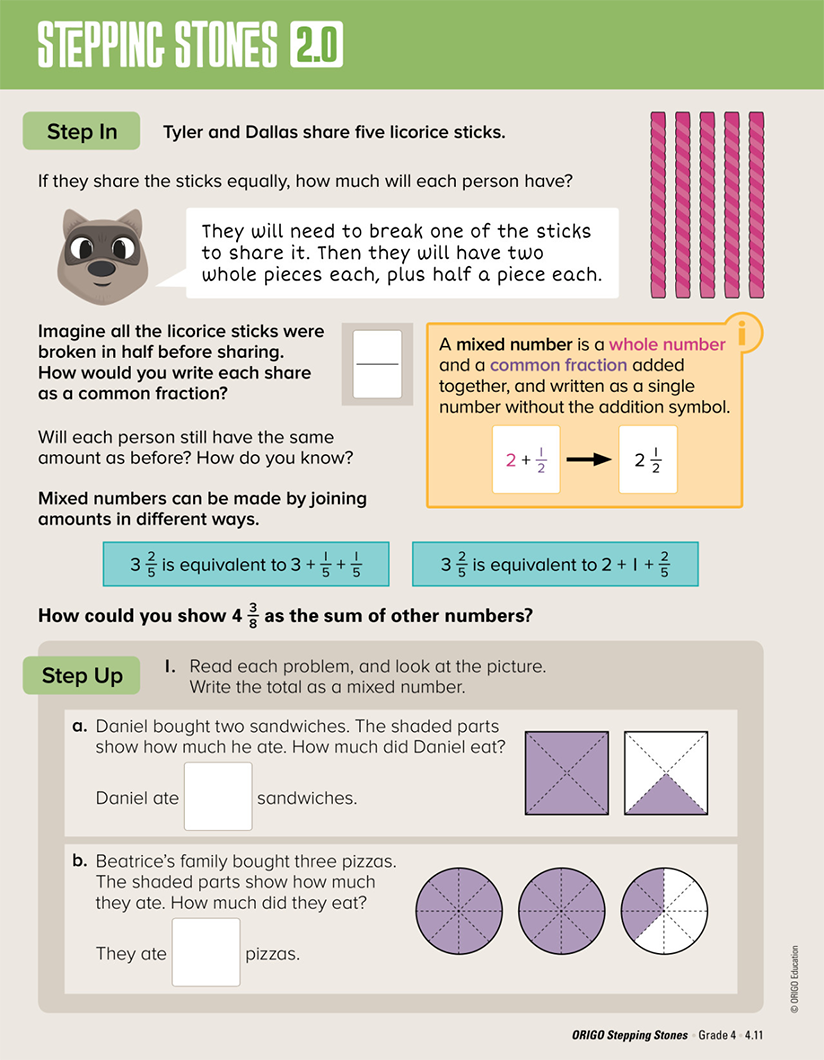
Take a moment to check out this typical ORIGO Stepping Stones lesson. Look at the way visual models are used and how students are asked to share their thinking and explain connections between those models.